2.7 Graphs of Rational functions

Graphing
In order to sketch the graph of a rational function in the form of:

where  and  have no common factors, follow this procedure:



1. - X-Intercepts: Find the solutions the equation: (setting the value of the numerator equal to 0). The real solutions to the equation represent the x-intercepts of the graph. (Keep track of any value that has a multiplicity greater than 1).



2. -Y-Intercept: Find and plot solution to  which represents the y-intercept on the graph.


3. -Vertical Asymptotes: Find the solutions to the equation  (setting the value of the denominator equal to 0). The real solutions represent the vertical asymptotes of the graph. (Keep track of any value that has a multiplicity greater than 1).



4. -Horizontal Asymptote:
a. If the degree of the numerator is less than the degree of the denominator                        , then the horizontal asymptote is y = 0.

b. If the degree of the numerator  is equal to the degree of the denominator , then the horizontal asymptote is the quotient of the leading coefficients.

c. If the degree of the numerator is greater than the degree of the denominator  there is no horizontal asymptote.


5. - Plot points if position of the curve cannot be found logically.



Example

Sketch the graph of:

X-Intercepts: Solve the equation:   to get x = 0 multiplicity 2, and x = 3. The X-Intercepts of the graph are located at (0,0) tangent to the x-axis & (3,0).

Y-Intercept: , so the y intercept is located at the point (0,0).

Vertical Asymptotes: Solve the equation  to get the vertical asymptotes of x = 2 multiplicity 2 & x = -2

Horizontal Asymptote: The degree of the numerator and the denominator are the same so the horizontal asymptote is equal to the quotient of the leading coefficients. Therefore, the horizontal asymptote is 

 or y = 3

Find a point the right of the asymptote x = 2

Plot the point (4,2) on the graph and use logic to draw the remaining curves. 

Holes

To graph the function

Graph the function:  and place a hole at (b, b - a)

Example
For the function: 

 , therefore is  indeterminate,

To graph this function, graph the line  and place a hole (represented with an empty circle) on the graph at (2,4) 

Oblique Asymptotes

If the degree of a numerator of a rational function is exactly one more than the degree of the denominator the function has a oblique asymptote.

To find the oblique asymptote use long division to divide the numerator by the denominator. 

Example

Use long division to get 

The oblique asymptote isbecause   as  

Info on Multiplicities

• If   than the curve at x-intercept (b, 0) will be tangent to the x-axis. 
• If  than both curves at the asymptote x = b head in the same direction. 

Helpful Links 

https://www.khanacademy.org/math/algebra2/rational-expressions-equations-and-functions/graphs-of-rational-functions/v/another-rational-function-graph-example

https://www.youtube.com/watch?v=y7KuUwydVZE

2.6 Rational Functions and Asymptotes

Rational Functions:
A rational function is a function that can be written as:

          

Where and  are polynomials and and  have no common factors


X Intercepts
To find X Intercepts you must find when
-You can do this through the factorization of the numerator

For Example: after factoring the numerator into  and

You find there is an X intercept at (-3, 0) and (3, 0)

Y Intercepts
To find Y Intercepts you must find what  is equal to
-You do this by plugging in 0 for every instance of X in a function
(If the denominator comes out as 0 then there is no Y Intercept, it is a Vertical Asymptote)
For Example:  after plugging in 0 for X you find that making there a Y Intercept at (0,-5)

Horizontal and Vertical Asymptotes:
An asymptote is defined as a line that continually approaches a given curve but never fully meets at any finite distance.

Vertical Asymptote:

To find a vertical asymptote you must find when  

-You can do this through the factorization of the denominator 

(Because where  it makes undefined)

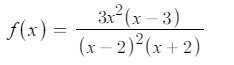
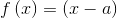
For example in the function   you can find the Vertical Asymptotes by factoring the denominator into  making two Vertical Asymptotes at and 

As you can see no line ever crosses the Asymptotes and  they only continue to approach to as X approaches 

Horizontal Asymptote:

When finding the horizontal asymptote you must find  

-You can do this by finding the end behavior of the given function

There are 3 things to check for when determining if there is a horizontal asymptote and if one exists what it is

1.) When the degree of the numerator is less than that of the denominator the Horizontal Asymptote is Y=0

Ex.)  

2.) When the degree of the numerator is equal to that of the denominator the Horizontal Asymptote is the ratio between their leading coefficients

Ex.)   

The Horizontal Asymptote here would be  Therefor the Horizontal Asymptote is Y=2

3.) When the degree of the numerator is greater than that of the denominator, no Horizontal Asymptote exists for that function

Ex.)  

*Note that the graph may cross the Horizontal Asymptote, yet their end behavior approaches the Horizontal Asymptote when one is present*