4.2 Trigonometric Functions: The Unit Circle

The unit circle is a method used to introduce trigonometric functions.

The unit circle is a circle on a x,y coordinate plane with its center on the origin with a radius of 1 unit, hence the name Unit Circle.

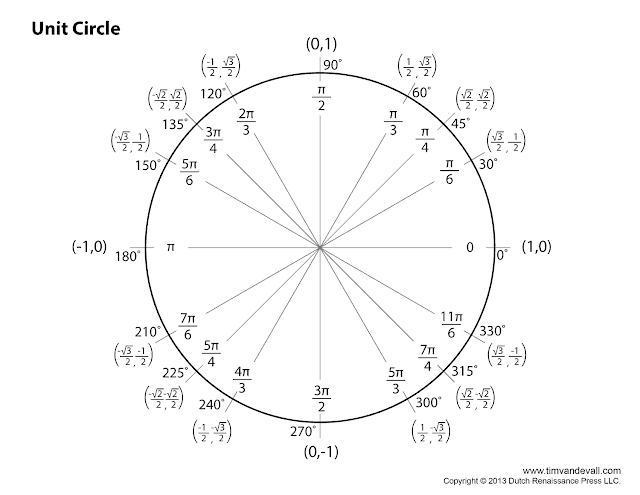
To find a circumference of a circle you use the equation C=2πr, therefore the unit circle has a circumference of 2π. You then can divide the circle into parts of 2π, such as 1/4π, to find the coordinates of a point with that arch length.

To find the x,y coordinates, remember special triangles and their relationship with the Pythagorean theorem.

Given that 360 degrees = 2π, then 30 degrees = 1/6π and 45 degrees= 1/4π

In a 30, 60, 90 triangle any side length will be proportional to each other in this way. This means that when a=1 then the coordinates of a point at 30 degrees will equal





In a 45, 45, 90 triangle any side length will be proportional to each other in this way. This means that when x=1 then the coordinates of a point at 45 degrees will equal 

 

 Using this principle, the entire unit circle looks like this. 

 In the unit circle

where



and 

Things to keep in mind...
 

•   

•  

 Also,

and




where c is equal to the measure of an angle of the unit circle, such as 2π. 

Above is a periodic function, meaning that 

In this function, when c is the smallest true value, c is known as the period of f.