Angles
Trigonometry means "measurement of triangles." Trigonometry deals with the relationships between the sides and angles of triangles.
An angle is determined by rotating a ray about its endpoint. The angle is the measure of rotation. It will have two sides, the initial side and the terminal side. The initial side is the starting position, and the terminal side is the position of the line after the rotation. The vertex is the endpoint of the ray.
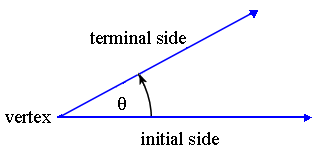
A standard position angle is an angle with its initial side on the positive x axis and its vertex on the origin.

Angles can be positive or negative. A positive angle has counterclockwise rotation, and a negative angle has clockwise rotation.
Angels can be coterminal, when they have the same initial and terminal sides.
Radian Measure
Angles can be measured in degrees or radians.
Definition of a radian: One radian is the measure of a central angle that intercepts an arc s equal in length to the radius r of the circle.
A radian is a measure of rotation just like degrees. A radian, however, is the amount of rotation needed to make the intercepted arc equal to the radius.
The circumference of a circle is equal to 2πr. Therefore, a central angle of one full revolution corresponds to an arc length of s = 2πsr. Because of this, we can conclude that 360° corresponds with 2π.
Because of this, when using radians, there is an easy formula to relate arc lengths to degree measure.
Cross multiply
Divide by 2π





No comments:
Post a Comment